Progression, in mathematics, is a sequence of related numbers or symbols called terms. The following examples illustrate three common kinds of progressions:
Arithmetic progression: 1,2,3,4,5,6, … and so on;
Geometric progression: 2,4,8,16,32, … and so on;
Harmonic progression: 1/2, 1/4, 1/6, 1/8, … and so on.
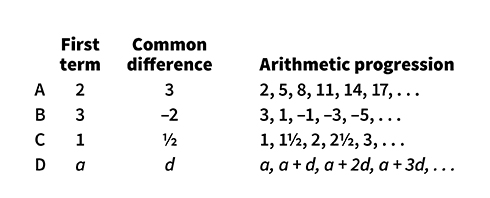
In each of these progressions, the terms after the first are formed in different ways. Each term of an arithmetic progression is formed by adding a quantity called the common difference to the previous term. In the example, the common difference is 1. Each term of a geometric progression is formed by multiplying the previous term by a quantity called the common ratio. In the example, the common ratio is 2. Each term of a harmonic progression is a fraction. The numerators are all 1’s and the denominators are formed like the terms of an arithmetic progression. In the example, the common difference of the denominators is 2.
Progressions are useful in solving many problems in science and business. For example, they simplify the calculation of compound interest (see Interest ). Mathematicians have developed formulas for finding the value of any term of a progression and for finding the sum of any number of terms.
Arithmetic progressions
may have various first terms and common differences, as shown below: