Cone, in geometry, is a solid figure whose base lies on a plane and is bounded by a closed curve called the directrix. The lateral surface (side) of the cone consists of all the line segments connecting points of the directrix to the cone’s vertex, a fixed point not on the plane of the base. When the directrix is a circle, the cone is a circular cone. If the line segment from the vertex to the center of the circle is perpendicular to the plane of the base, the cone is a right circular cone. When a right circular cone is intersected by a plane that does not contain the vertex, a curve called a conic section is formed. Circles, ellipses, hyperbolas, and parabolas are conic sections.
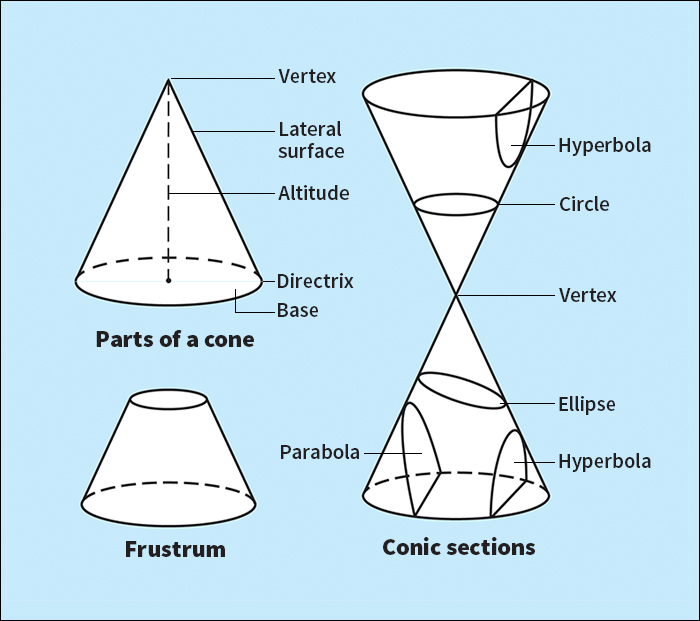
The altitude of a cone is the perpendicular line segment from the vertex to the plane of the base. The length of the altitude is the height of the cone. The volume (V) of a cone can be found by using the formula: V = 1/3 Bh. In the formula, B stands for the area of the base and h for the height of the cone. If the base of the cone is a circle, then B = πr 2, where r is the radius of the circle. The formula for volume can then be written: V = 1/3πr 2 h. An approximate value for π (pi) is 3.1416.
In a right circular cone, all the line segments from the vertex to the directrix have the same length, called the slant height. The lateral surface area (L) of a right circular cone can be calculated with the formula: L = πrs. In this formula, r stands for the radius of the base and s stands for the slant height. The total surface area of the cone equals the lateral surface area plus the base area.
If a plane parallel to the base of a cone passes between the vertex and the base, the cone is divided into a smaller cone and a solid figure called a frustum. The volume of the frustum equals the volume of the original cone minus the volume of the smaller cone.
