Cuisenaire << `kwihz` uh NAIR >> Method is a teaching system designed to help students discover basic mathematical principles by themselves. The method uses rods of 10 different colors and lengths that are easy to handle. By using the rods, students can prove numerical relationships and understand principles of proportion. Students can also use the colored rods in learning addition, subtraction, multiplication, division, factoring, and fractions.
The rods help the student understand mathematical principles rather than merely memorize them. For example, two white rods placed end to end are the same length as a red rod. The red rod then stands for the number 2 if the white rod represents 1. A red rod and a white rod placed end to end are as long as a light-green rod. The light-green rod then stands for 3, because it is as long as three white rods. The rods can also be used to represent different sets of numbers. For example, if the white rod is assigned a value of 3, the red rod becomes 6.
Students can discover many facts about mathematical relationships by working with the rods. For example, three red rods placed end to end are as long as one dark-green rod. The dark-green rod is two-thirds as long as a blue rod. Students can see that the three red rods equal two-thirds of a blue rod.
The rods also can be used to teach such elementary arithmetic properties as the associative, commutative, and distributive properties. For instance, to help students understand that 3 X 2 = 2 X 3, an example of the commutative property, two light-green rods can be placed on top of three red rods.
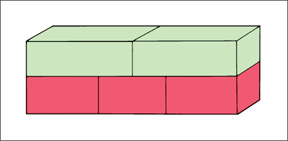
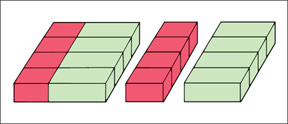
To show that 4 X (2 + 3) = (4 X 2) + (4 X 3), an example of the distributive property, a set of four red rods and four light-green rods can be split into two sets. One of the two sets would have four red rods. The other would have four light-green rods.
Schoolteachers use the Cuisenaire Method in the United States, Canada, and parts of Europe. Emile-Georges Cuisenaire, a schoolteacher from Thuin, Belgium, developed the method.
