Golden section, also called the divine proportion, is the division of a line segment in such a way that the ratio of the whole segment to the larger part is equal to the ratio of the larger part to the smaller part. The ratio is approximately 1.61803 to 1.
A rectangle whose length and width correspond to this ratio is called a golden rectangle. Rectangles that look like a golden rectangle are more pleasing to the eye than other rectangles, though no one knows why. Many golden sections and golden rectangles appear in famous paintings, sculpture, and architecture. Buildings that incorporate golden sections and rectangles include the Parthenon, constructed in Athens in the 400’s B.C., and buildings designed in the 1900’s by the French architect Le Corbusier.
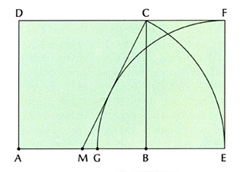
The figure with this article shows how to construct a golden rectangle and a golden section. First draw a square ABCD. Then, locate the midpoint M of side AB. Next, use a compass to extend AB to a point E so that ME = MC. Rectangle AEFD is a golden rectangle. To divide AB according to the golden section, use a compass to find point G on AE so that EF = EG.