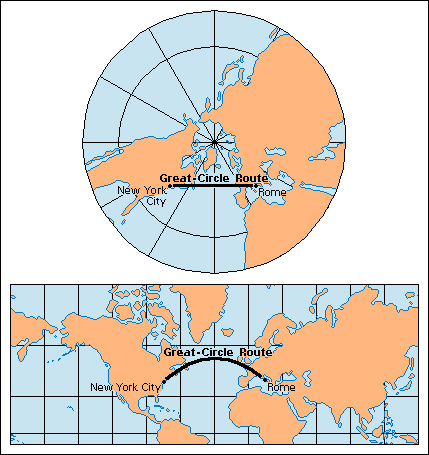
Great-circle route is the shortest, most direct route between two points on the earth’s surface. A great circle is any circle that divides the globe into equal halves. Its length is the same as that of the equator. On most flat maps, a straight line appears to be the shortest distance between two places. A great-circle route often appears as a longer curve. But maps are not true pictures of the surface of the earth. Maps are flat, but the earth is a sphere. The shortest distance between any two points on the earth can be found easily only on a globe. The shortest distance lies along the great circle passing through the two points. A special kind of map called a gnomonic projection shows a great-circle route as a straight line.
To follow a great-circle route exactly, a ship must constantly change the compass direction in which it is heading. A ship’s navigator would find it hard to follow a rapidly changing course. Instead, the navigator can plot a course with a series of connected lines, each line following a constant direction. These lines, called rhumb lines, join selected points along the great-circle route. By following the compass directions that are indicated by the rhumb lines, the ship can sail a course that is close to the shortest possible route. Airplanes can follow great-circle routes more easily than can ships. Many airplanes use a method of navigation known as inertial guidance, which allows aircraft to follow great-circle routes accurately. Ballistic missiles also use inertial guidance systems to follow great-circle routes (see Inertial guidance ).
In 1537, Pedro Nunes, a Portuguese navigator, wrote about the advantages of great-circle-route navigation. Most ships did not sail great-circle routes until the 1800’s, when navigation improved. Since the early 1900’s, great-circle routes have determined the major air routes.
