Logarithms

Logarithms, << LAWG uh rihth uhmz or LOG uh rihth uhmz, >> are numbers that are known in algebra as exponents. Exponents are used to express repeated multiplications of a single number. For example, 2 X 2 X 2 can be written 2³. In the equation 2³ = 8, 3 is the exponent and 2 is the base. Stated in terms of logarithms, 3 is the logarithm of the number 8 to the base 2. This statement can be written as log₂8 = 3. The equation log₂8 = 3 is another way of expressing 2³ = 8. In general, if b^x = p, then x = log_bp.

Suppose you want to calculate the number of ancestors you have in each of three previous generations. You have 2 parents, so you have 2 ancestors in the first generation. This calculation can be expressed as 2¹ = 2. Each of your parents has 2 parents, so you have 2 X 2 = 2² = 4 ancestors in the second generation. Each of your grandparents has 2 parents, so you have 4 X 2 = 2 X 2 X 2 = 2³ = 8 ancestors in the third generation. The calculation continues in this pattern. In which generation do you have 1,024 ancestors? That is, for which exponent x is it true that 2^x = 1,024? You can find the answer by multiplying 2 by itself until you reach 1,024. But if you know that log₂1,024 = 10, you know the answer is 10.

The laws of logarithms

Because logarithms are exponents, the properties of exponents apply to them. The following equations show some of the important properties of exponents:

(1) 3² X 3³ = 3 X 3 X 3 X 3 X 3 = 3⁵, so 3² X 3³ = 3^{2 + 3}. Thus, b^x X b^y = b^{x + y}.

(2) 3⁵ ÷ 3² = (3 X 3 X 3 X 3 X 3) ÷ (3 X 3) = 3³, so 3⁵ ÷ 3² = 3^{5 – 2}. Thus, b^x ÷ b^y = b^{x – y}.

(3) (2³)² = 2³ X 2³ = 2^{3 + 3} = 2⁶, so (2³)² = 2^{3 X 2}. Thus, (b^x)^y = b^xy.

These properties of exponents can be restated as properties of logarithms:

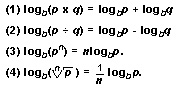
To show that the first property is true, let log_bp = x and log_bq = y. Then p = b^x and q = b^y. So p X q = b^x X b^y and p X q = b^{x + y}. Since p X q = b^{x + y}, log_b(p X q) = x + y. Therefore, log_b(p X q) = log_bp + log_bq. The other three properties can be derived in a similar way.

Using logarithms

Multiplication.

To multiply two numbers using logarithms, look up the logarithms of the two numbers in a table. Add these logarithms to get the logarithm of the product of the two numbers. Then, using the table again, find the number whose logarithm is the logarithm of the product. This is the product of the two numbers.

Division.

To divide one number by another, look up the logarithms of the two numbers in a table. Subtract the logarithm of the denominator from the logarithm of the numerator. Then, using the table again, find the number whose logarithm is the same as the logarithm found by this subtraction. This number is the desired quotient.

Raising a number to a power.

To raise a number to a power, look up the logarithm of the number in a table. Multiply this logarithm by the exponent of the power. Then, using the table again, find the number whose logarithm is the same as the logarithm found by this multiplication. This number is the desired power of the first number.

Finding a root.

To find a root of a number, look up the logarithm of the number in a table, and divide this logarithm by the index of the root. Then, using the table again, find the number whose logarithm equals the number found by the division. This is the desired root of the number. See Root ; Square root .

Kinds of logarithms

Common logarithms.

Any positive number, other than 1, can serve as a base for logarithms. However, 10 is the most convenient base because the most common number system is based on 10. Logarithms to the base 10 are called common logarithms.

The common logarithms of two numbers that have the same sequence of digits, such as 247 and 2.47, differ only by an integer (positive or negative whole number). For example, 247 = 100 X 2.47 = 10² X 2.47. Therefore, log₁₀247 = log₁₀ 10² + log₁₀ 2.47 = 2 + log₁₀ 2.47. Thus, the common logarithms of 247 and 2.47 differ only by the whole number 2. In fact, to four decimal places, the common logarithm of 247 is 2.3927 and that of 2.47 is 0.3927.

Because 247 lies between 100 and 1,000, that is between 10² and 10³, log₁₀247 lies between log₁₀ 10² and log₁₀ 10³. That is, the common logarithm of 247 lies somewhere between 2 and 3. Thus, the integer part of log₁₀247, or of any other common logarithm, can be determined mentally.

In common logarithms, the integer part is called the characteristic, and the decimal part is called the mantissa. For a given sequence of digits, a shift in the position of the decimal point changes the characteristic, but not the mantissa. Because the characteristic can be determined mentally, tables of common logarithms list only mantissas.

Natural logarithms.

Mathematicians and scientists use natural logarithms in their work. In the system of natural logarithms, the base is the number e = 2 + ¹/₂ + ¹/_2×3 + ¹/_2x3x4 … = 2.71828… . Natural logarithms are useful in calculus because many important formulas take their simplest possible forms using them.

History

John Napier, a Scottish mathematician, published the first discussion and table of logarithms in 1614 (see Napier, John ). Jobst Burgi of Switzerland independently discovered logarithms at about the same time. In the early 1600’s, Henry Briggs of England introduced logarithms to the base 10 and began constructing a 14-place table of common logarithms. Adriaen Vlacq of the Netherlands completed Briggs’s work.

The Briggs-Vlacq tables remained in use until 20-place common logarithm tables were calculated in Britain between 1924 and 1949. Today, computers and electronic calculators have eliminated the need to use logarithms for computation. However, logarithms continue to be important for theoretical purposes.